

We recommend using aĪuthors: Lynn Marecek, Andrea Honeycutt Mathis Use the information below to generate a citation. Then you must include on every digital page view the following attribution: If you are redistributing all or part of this book in a digital format, Then you must include on every physical page the following attribution: If you are redistributing all or part of this book in a print format,

Want to cite, share, or modify this book? This book uses the
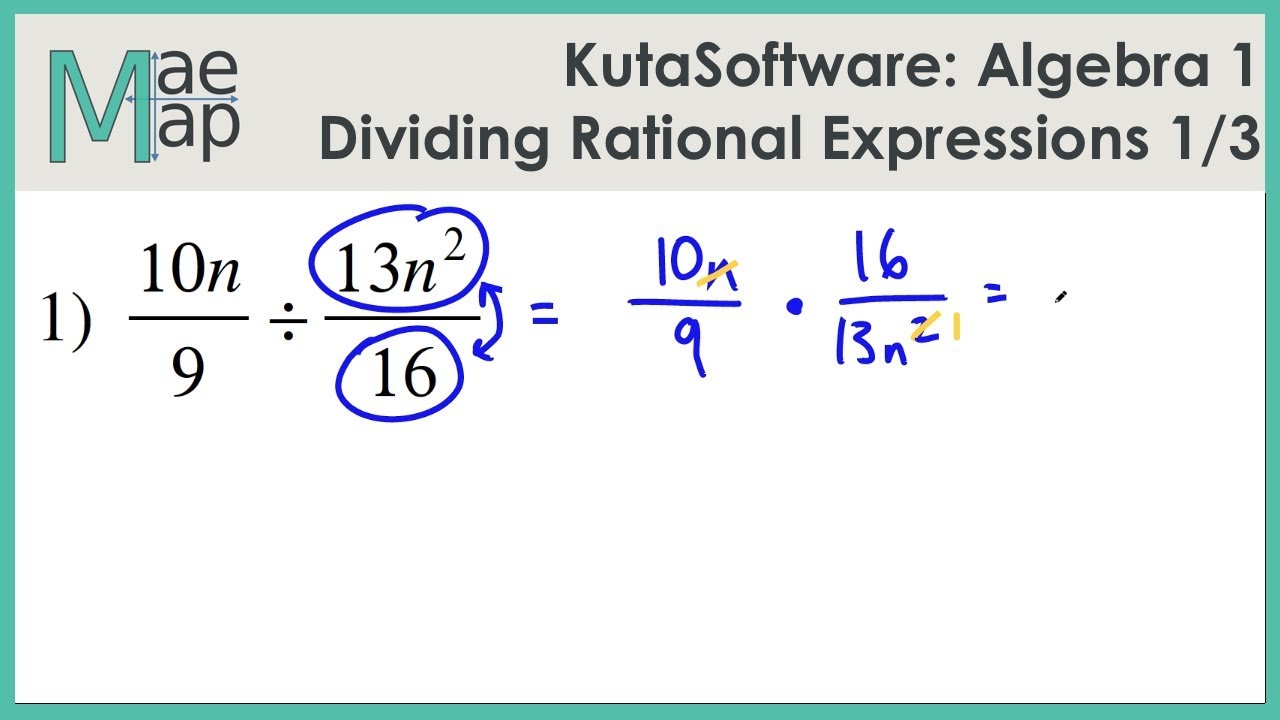
Together you can come up with a plan to get you the help you need. See your instructor as soon as possible to discuss your situation. You need to get help immediately or you will quickly be overwhelmed. …no - I don’t get it! This is critical and you must not ignore it. Is there a place on campus where math tutors are available? Can your study skills be improved? Whom can you ask for help?Your fellow classmates and instructor are good resources. It is important to make sure you have a strong foundation before you move on. Math is sequential - every topic builds upon previous work. This must be addressed quickly as topics you do not master become potholes in your road to success. What did you do to become confident of your ability to do these things? Be specific! Congratulations! You have achieved your goals in this section! Reflect on the study skills you used so that you can continue to use them. Ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section. Did you get the same answer you got in part ⓐ ? Why or why not? Self Check ⓒ Evaluate your answer to part ⓑ when x = 5. ⓑ Divide x 2 − 1 x ÷ ( x + 1 ) x 2 − 1 x ÷ ( x + 1 ) and explain all your steps. Ⓐ Divide 24 5 ÷ 6 24 5 ÷ 6 and explain all your steps. That way, when we solve a rational equation for example, we will know whether the algebraic solutions we find are allowed or not. So before we begin any operation with a rational expression, we examine it first to find the values that would make the denominator zero. In order to avoid dividing by zero in a rational expression, we must not allow values of the variable that will make the denominator be zero. When we work with a numerical fraction, it is easy to avoid dividing by zero because we can see the number in the denominator. Multiplying rational expressions is similar to multiplying fractions. The numerator of a rational expression may be 0-but not the denominator. Multiplying and Dividing Rational Expressions (continued). If the denominator is zero, the rational expression is undefined. Determine the Values for Which a Rational Expression is Undefined
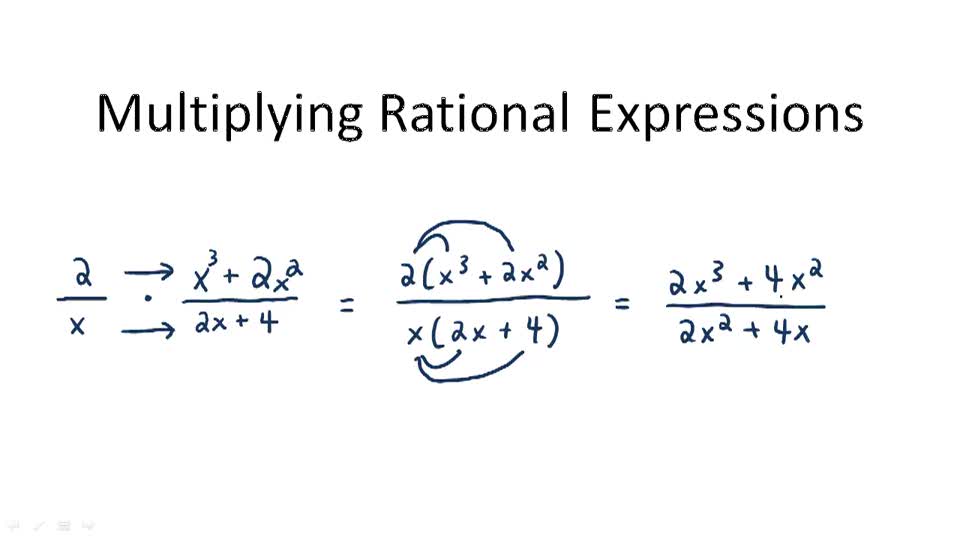
We will simplify, add, subtract, multiply, divide and use them in applications. We will do the same operations with rational expressions that we did with fractions. Since a constant is a polynomial with degree zero, the ratio of two constants is a rational expression, provided the denominator is not zero. Notice that the first rational expression listed above, − 24 56 − 24 56, is just a fraction. ( x + 5 ) ( x − 1 ) ( 2 x − 1 ) 3 ( x + 6 ) ( x + 5 ) Cancel common factors to simplify. ( x + 5 ) ( x − 1 ) ( 2 x − 1 ) 3 ( x + 6 ) ( x + 5 ) Multiply numerators and denominators. ( x + 5 ) ( x − 1 ) 3 ( x + 6 ) ⋅ ( 2 x − 1 ) ( x + 5 ) Factor the numerator and denominator.


 0 kommentar(er)
0 kommentar(er)
